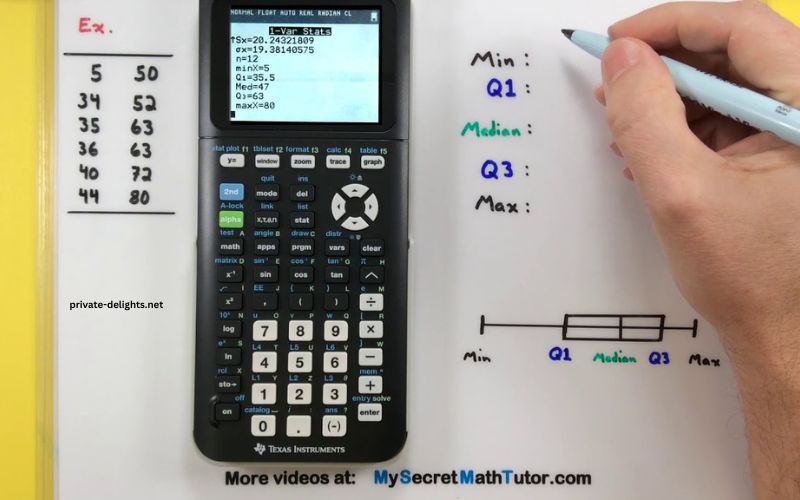
The 5-number summary is a fundamental tool in descriptive statistics, providing a concise summary of a data set through five key values: the minimum, first quartile (Q1), median (Q2), third quartile (Q3), and maximum. This summary offers a snapshot of the data distribution, helping to identify patterns, spread, and potential outliers.
Components of the 5-Number Summary
- Minimum: The smallest value in the data set.
- First Quartile (Q1): The median of the lower half of the data set, representing the 25th percentile.
- Median (Q2): The middle value of the data set, dividing it into two equal halves, representing the 50th percentile.
- Third Quartile (Q3): The median of the upper half of the data set, representing the 75th percentile.
- Maximum: The largest value in the data set.
Importance of the 5-Number Summary
The 5-number summary is valuable for several reasons:
- Simplicity: It reduces a complex data set into five easy-to-understand values.
- Robustness: It is less affected by outliers compared to measures like the mean.
- Visualization: It forms the basis for box plots, which visually depict data distribution and identify outliers.
Calculating the 5-Number Summary
Step-by-Step Process
- Order the Data: Arrange the data points in ascending order.
- Identify the Minimum and Maximum: The smallest and largest values, respectively.
- Calculate the Median (Q2):
- For an odd number of data points, it is the middle value.
- For an even number of data points, it is the average of the two middle values.
- Determine the First Quartile (Q1):
- It is the median of the lower half of the data set (excluding Q2 if odd).
- Determine the Third Quartile (Q3):
- It is the median of the upper half of the data set (excluding Q2 if odd).
Example Calculation
Consider the data set: 6, 2, 9, 4, 7, 3, 8, 5, 1.
- Order the Data: 1, 2, 3, 4, 5, 6, 7, 8, 9.
- Minimum: 1.
- Maximum: 9.
- Median (Q2): 5 (middle value).
- First Quartile (Q1):
- Lower half: 1, 2, 3, 4.
- Median of the lower half: Q1=2+32=2.5Q1 = \frac{2 + 3}{2} = 2.5.
- Third Quartile (Q3):
- Upper half: 6, 7, 8, 9.
- Median of the upper half: Q3=7+82=7.5Q3 = \frac{7 + 8}{2} = 7.5.
The 5-number summary is: 1, 2.5, 5, 7.5, 9.
Using a 5-Number Summary Calculator
A 5-number summary calculator automates the calculation process, making it quicker and more accurate, especially for large data sets. These calculators are typically available online and in statistical software.
How the Calculator Works
- Data Input: Enter the data set into the calculator.
- Processing: The calculator sorts the data, identifies the minimum and maximum, and calculates the quartiles and median.
- Output: The calculator displays the five critical values: minimum, Q1, median, Q3, and maximum.
Features of a Good 5-Number Summary Calculator
- User-Friendly Interface: Easy data input and clear presentation of results.
- Accuracy: Reliable calculations that handle large and complex data sets.
- Visualization: Some calculators provide graphical representations, such as box plots.
- Additional Statistics: Advanced calculators might offer extra statistics like the interquartile range (IQR), mean, standard deviation, and outlier detection.
Practical Applications of the 5-Number Summary
Education
In education, teachers use the 5-number summary to analyze test scores, providing insights into student performance and identifying those who may need additional help or recognition.
Finance
Financial analysts use it to summarize stock prices, returns, and other financial metrics, helping to identify trends and make informed investment decisions.
Healthcare
Healthcare professionals analyze patient data, such as blood pressure readings or cholesterol levels, to understand the distribution and identify any extreme values that require attention.
Research
Researchers use the 5-number summary to present data succinctly in fields such as environmental science, social studies, and experimental research.
Limitations of the 5-Number Summary
Despite its usefulness, the 5-number summary has some limitations:
- Loss of Detail: It reduces the data to five values, potentially overlooking nuances in the data set.
- Outliers: While it can indicate the presence of outliers, it does not provide detailed information about them.
- Symmetry Assumption: It assumes a symmetric distribution around the median, which may not be accurate for all data sets.
Conclusion
The 5-number summary is a fundamental tool in descriptive statistics, providing a quick and effective way to understand data distribution and central tendency. Whether you are an educator, financial analyst, healthcare professional, or researcher, mastering this tool can greatly enhance your data analysis capabilities. With the help of 5-number summary calculators, the process becomes even more accessible, ensuring accurate and efficient data summarization. Despite its limitations, the 5-number summary remains an invaluable tool for making sense of complex data sets and drawing meaningful insights.